Coastlines Investigation
 Lesson 1: Measuring the Coastline of
Nahant
Lesson 1: Measuring the Coastline of
Nahant
Originally, I planned to have students measure the coastline of their home town of Chelsea, MA. After inspecting several maps, I realized that the waterfront of Chelsea along the Chelsea Creek is a smooth, manmade wharf not a ragged, natural coastline. Close by is the peninsula of Nahant. Nahant's jagged coastline is more interesting for an investigation of fractals.

Using calipers or a pencil compass and a blow-up of a Boston area map, students "walked" around the peninsula of Nahant using different step sizes. Because of the jagged coastline the distance "walked" increases as the step-size decreases. As the data table to the right shows, cutting the step size in half more than doubles the number of steps needed to "walk" the coastline.
The rate of increase in steps taken, can be determined by plotting number of steps versus step size on a log-log graph. The slope of this graph corresponds to the exponential increase in steps as the step-size is reduced. This exponent also corresponds to the fractional dimension of the coastline. If the coastline is smooth, the slope or exponential increase will be close to one - a linear or one-dimensional coastline. If the coastline is extemely jagged the slope or exponential increase will be close to two.
We found that the coastline of Nahant has a fractal dimension of 1.2. This would suggest that the coast of Nahant is not very jagged.
 Lesson 2: Using the program
Fractal Coastline
Lesson 2: Using the program
Fractal Coastline
Pairs of students working on a Macintosh Color Classics explored the tools of the fractal coastline program. Students were to make a coastline, measure the length of the coastline with different size steps or rulers, graph the number of steps versus step size, and determine the fractal dimension from the slope of the graph. With a little guidance students were able to use the graphical interface and pull down menus of "Fractal Coastline" to complete these steps.
After successfully completing this exploration, we setup a classroom investigation. The coastline is randomly produced based on parameters set by the user. For our investigation all groups set the starting points at three and the roughness at 0.5. Each group used a different number of iterations to make their coastline. Once each group had completed the measuring and graphing of their results, we made a data table of number of iterations versus fractal dimension of the coastline. As the number of iterations is increased the jaggedness of the coastline increases, increasing the fractal dimension of the coastline.
Lesson 3: The Meaning of Dimension
The Coastline Investigation gives students a chance to get their feet wet with fractals. But after completing the investigation many students are still confused about what a fractal is and what the slope of the log-log graphs represents. The meaning of the slope of the log-log graph is clarified by investigating a disc and a line. Students associate these objects with two and one dimensions, respectively. Using the new techniques of plotting number of steps (or number of squares for the the disc) versus step size (or size of square for the disc), and seeing that the resulting slope is two and one respectively gives students a context for the investigation they have completed.
The question 'what is a fractal?' requires students to see and
explore alot more examples of patterns in nature. This question is
partially answered by the next investigation which explores the
sierpinski triangle. Another approach might be to begin with a
qualitative investigation of a variety of different natural objects and
images. These objects and images could be plants, breaking ocean waves,
mountain ranges, ink stains, wrinkled or torn paper. Students would
discuss and write down qualitative observations about one or more of
these objects. The following questions could prompt their thinking: How
does the object fill space? Is its use of space dense or sparse? Are
its edges smooth or jagged? What is similiar throughout different parts
of the object? What is random or different throughout different parts
of the object? How does the whole object, compare with individual parts
of the object? What geometric shapes do you see in the object: circles,
lines, ovals, spheres?
Each stage of the project requires different lab groups to complete
different tasks: connecting and measuring resistance of each stage of
the network, collecting and organizing resistance data. The students
were given a table
with assignments and instructions for each phase of the project.
Each group started with nine 100 ohm resistors and a circuit board.
Sierpinski Resistor Networks Investigation
 The objective of this project is to compare the equivalent resistance of a resistor network connected as a seirpinski triangle with other
resistor networks: series, parallel, and series-parallel. The project
is conducive to a collaborative learning experience. Each lab group is
responsible for building and measuring the equivalent resistance of one
subunit of the larger sierpinski resistor network.
The objective of this project is to compare the equivalent resistance of a resistor network connected as a seirpinski triangle with other
resistor networks: series, parallel, and series-parallel. The project
is conducive to a collaborative learning experience. Each lab group is
responsible for building and measuring the equivalent resistance of one
subunit of the larger sierpinski resistor network.
Building the Sierpinski Resistor Network
 Stage One of the Network
Stage One of the NetworkNine lab groups put together a
stage one network. The resistance
is measured from two of the
outer triangle nodes. The average
resistance of the stage one networks
was 129 ohms.
 Stage Two of the Network
Stage Two of the NetworkThree lab groups collaborated to
put together a stage two network.
The average resistance of stage
two was 213 ohms.
 Stage Three of the Network
Stage Three of the NetworkFinally each groups network
contributed to the stage three
network. The resistance of
the stage three network was
378 ohms.
Comparison of Resistor Networks
The students compared the results of the sierpinski resistor network with calculated values for series, parallel, and series-parallel networks. This is the graph and conclusion they produced:
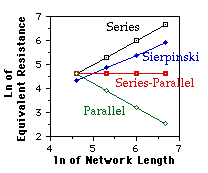
We plotted the natural logarithm of the equivalent resistance versus the natural logarithm of the resistance of one leg of the network. the slope indicates the exponential increase in equivalent resistance for each network.
The series circuit is one-dimensional. The equivalent resistance increases linearly (the slope = 1.0). The series-parallel circuit is two dimensional. The equivalent resistance of the series-parallel is constant (the slope is zero). We are not sure how to define the dimension of the parallel circuit, but its equivalent resistance decreases linearly (the slope = -1.0). The equivalent resistance of the Sierpinski Resistor Network increases at an exponential rate of 0.7609.
In summary, as the fractal dimension of the network increases, the equivalent resistance decrease. As the network increases in fractal dimension more connections exist between the resistors. Each path or connection decreases the networks resistance.