|
Your objective is to:
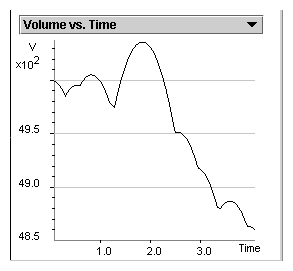
Recognize the effect of molecular collisions with the piston on
the piston's position.
You will be able to:
Predict what happens to the position of the piston when the
external pressure is greater than the internal pressure of the
gas.
Explain why the position of the piston fluctuates when the external and
internal pressures are approximately equal.
Describe gas pressure in terms of molecular collisions.
State the relationship between frequency of collision and the volume of a
given gas sample.
|