HandsOn
26 - Liesegang Experiment
I.
Pre-Lab
Figure
6.6: Multiple parallel Liesegang rings in silica gel.
Figure
6.7: Circular Liesegang rings in a petri dish of agar gel.
There
are clear similarities between the Liesegang experiment and the
diffusion tube experiment. In both experiments:
-
the reaction is dependent on the diffusion of the reactants,
-
the reaction is between two different molecules and results in
the precipitation of a salt, and
-
in both reactions the chemical reactants diffuse along a cylinder.
There
are, however, some differences between the two diffusion experiments:
-
one of the reactants, the potassium chromate, is initially uniformly
distributed along the cylinder in the Liesegang experiment,
-
the diffusion takes place in a gel as opposed to air, and of course
-
multiple disks appear instead of a single disk.
|
Q6.15: Can you think of other differences and similarities
between the diffusion chamber and Liesegang experiments?
|
|
|
Q6.16: Can you account for the appearance of multiple
parallel disks in Figures 6.6 and 6.7? Think of what happens
as the copper sulfate diffuses into the gel? Will a precipitate
of copper chromate appear? If so, what will that do to
the local concentration of chromate ion? What will be
the consequence for diffusion of chromate ion to or from
nearby regions? What happens to the local concentration
of the copper ions diffusing through the gel? What happens
next? |
|
|
Q6.17: Can you make a guess as to how long you may have to wait to
observe a pattern? Hint: A generalization of the result obtained in
Appendix 6-A to cases when randomness of steps is caused by collisions
with other particles turns out to be
áx2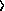 avg = 2Dt, avg = 2Dt, |
|
where
the proportionality constant D is called the diffusion constant, and
its value varies from material to material. The value of the diffusion
constant for copper sulfate in the gel is roughly what it is in water,
on the order of 10-6 cm2/sec. The preceding
equation is usually called the diffusion equation.
|
|
II. Experiment
For this experiment,
you will need the following materials:
- Glacial acetic
acid (17.4 M)
- Potassium Chromate
(K2CrO4)
- Sodium Silicate
solution (commercial water glass) with specific gravity 1.38-1.42 g/ml
- Copper Sulfate
crystals
- distilled water
- one 2 dram
vial (7.4 ml), actually holds up to 10 ml
- two 50 ml beakers
- 5 ml pipette
calibrated in 0.1 ml units
- 0.1 ml pipette
calibrated in 0.01 ml units
In the following,
it is assumed that the experiment will be done with approximately 8 ml of gel.
For other sizes, scale the ingredients proportionately. Leave space at the top
of the vial for the copper sulfate.
1. Make 4 ml of sodium silicate solution by adding 0.6 ml of sodium silicate
(specific gravity 1.38-1.42) to 3.4 ml of distilled water. This produces a solution
with specific gravity 1.06.
2. Make 4 ml of acetic acid solution by adding 0.09 ml of glacial acetic acid
to 3.9 ml of distilled water. To this add 0.19 g of potassium chromate. Make
this solution in the vial.
3. Pour the sodium silicate solution made in Step 1 into the vial containing
the acetic acid solution of Step 2. Put on the cap (quickly) and mix all the
reagents by inverting the vial about 10 times. Don't agitate the vial - this
will introduce air bubbles into the gel and may distort the pattern.
4. Leave the gel in a safe place where it will not be moved or shaken. The
solution will gel in 5 to 20 minutes. Let it harden for several hours if possible.
5. After hardening, add between 0.5 g and 1.0 g of copper sulfate crystals
to the top of the gel. Try to cover the surface uniformly.
6. You will start seeing patterns after roughly 24 hours. The pattern will
continue forming for one and a half weeks to two weeks.
7. Observe the pattern formed. Record the time it takes for portions of the
pattern to develop. Measure the distances between portions of the pattern.
|
Q6.18: Compare your predicted pattern with what you observed. Is the
symmetry what you expected? Refine your speculation about the source
of the multiple rings. |
|
|
Q6.19: Did the pattern take as long to develop as you expected? Does
the diffusion equation above provide an approximate relation between
the time t for a single ring to form? |
|
Previous: 6.2
- Periodic Precipitation: Liesegang Rings


